We have eleven (11) radical equations lined up for you here. Each one is a bit different from the last. Dive in, and remember, it’s all about taking it one problem at a time. You’ve totally got this!
Problem 1: Solve the radical equation below.
Since the square root of a real number must be positive, then this radical equation [latex]\color\text[/latex] have a real solution. Notice the right-hand side is negative, that is, [latex]\color-1[/latex].
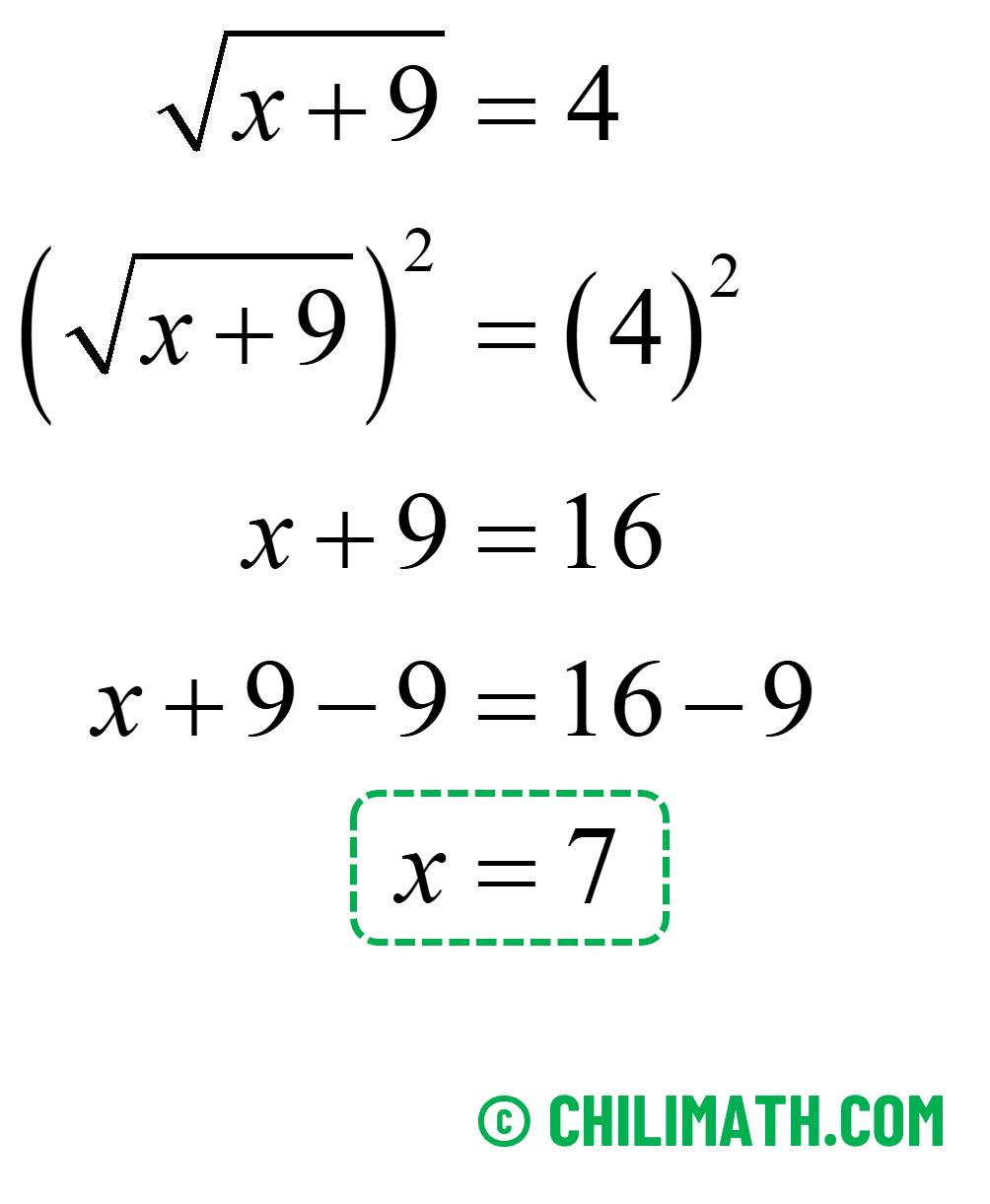
Problem 2: Solve the radical equation below.
Start by squaring both sides of the equation. Then subtract both sides by [latex]9[/latex].

Verify the answer by substituting it back to the original radical equation to check if it yields a true statement.

Therefore, the solution is [latex]7[/latex].
Problem 3: Solve the radical equation below.
Add [latex]5[/latex] to both sides of the equation. Square both sides to eliminate the square root. Subtract [latex]1[/latex] from both sides. Finally, divide both sides by [latex]-3[/latex].

Validate [latex]x=-1[/latex] from the original radical equation.

Therefore, [latex]x=-1[/latex] is a solution.
Problem 4: Solve the radical equation below.
[latex]\sqrt x \,- \,1 = 9\, – \,2x[/latex]
Start by isolating the square root term. Then, square both sides to eliminate the square root. Expand and simplify the resulting expression to form a quadratic equation. Factor out the trinomial to find the potential solutions. Finally, verify each solution by substituting it back into the original equation to ensure it is correct. The valid solution is identified through this verification process.

I will leave it to you verify [latex]x=4[/latex] and [latex]x =<\Large< <25 \over 4>>>[/latex] by substituting each back to the original radical equation. You should find out that the only valid answer is [latex]x=4[/latex].
Problem 5: Solve the radical equation below.
To solve the equation, first cube both sides to eliminate the cube root. Simplify the resulting equation and solve for [latex]x[/latex]. Verify the solution by substituting it back into the original equation to ensure it is correct. If it checks out, the solution is valid.


Therefore, [latex]x=11[/latex] is a solution to the given radical equation.
Problem 6: Solve the radical equation below.
To solve the radical equation, square both sides to eliminate the square roots. Multiply the binomials on the left side which resulting to quadratic equation. Simplify the terms, then use the factoring to find the solutions. Verify each solution in the original equation and identify the valid ones.


Note that the only solution is [latex]x =<\Large< <5 \over 6>>>[/latex] which makes [latex]x=-1[/latex] an extraneous solution.
Problem 7: Solve the radical equation below.
To solve the equation, first square both sides to eliminate the square root, then expand the right side. Move all terms to one side to form a quadratic equation and simplify it. Use the factoring method to solve for [latex]x[/latex]. Verify each solution by substituting it back into the original radical equation to check for validity. Determine which solutions satisfy the original equation and conclude with the valid one.

Here, [latex]x=3[/latex] is not a valid solution.
The only solution is [latex]x=7[/latex].
Problem 8: Solve the radical equation below.
[latex]\sqrt \,- \,5x \,- \,7> = x + 1[/latex]
To solve the given equation, we first square both sides to eliminate the square root, then simplify the resulting equation. Next, we bring all terms to one side to set the equation to zero and solve the resulting quadratic equation using the factoring method. We then check each solution in the original equation to ensure they do not produce extraneous solutions. After verification, we determine that both solutions satisfy the original equation.


Thus, the solutions are [latex]x=8[/latex] and [latex]x=-1[/latex].
Problem 9: Solve the radical equation below.
We start by squaring both sides of the equation to eliminate the square roots. After simplifying, we isolate the square root term once more on the right side of the equation. Then, we square both sides again to remove the square root term, resulting in a quadratic equation. We simplify this quadratic equation and proceed to solve it by factoring.


Lastly, we verify the obtained solutions by substituting them back into the original equation.
Therefore, the solutions are [latex]x=5[/latex] and [latex]x=1[/latex].
Problem 10: Solve the radical equation below.
Get rid of the square roots by squaring both sides of the equation. We will have to do it twice. Eventually, the quadratic term [latex]x^2[/latex] will be eliminated leaving a linear equation to solve which is very easy to address.

Verify that [latex]x=7[/latex] is a solution to the radical equation.
Problem 11: Solve the radical equation below.
Square both sides of the equation. Simplify, then square both sides again to eliminate the square roots. Next, move all terms to the left side to set the right side equal to zero. Factor the trinomial on the left into two binomials. Lastly, set each binomial equal to zero to solve for [latex]x[/latex].

We need to verify our solutions from the original radical equation.

We should find out that [latex]x =<\Large< <7 \over 5>>>[/latex] is true while [latex]x=-5[/latex] is false.
Therefore, the only solution is [latex]x =<\Large< <7 \over 5>>>[/latex].
You might also like these tutorials:
Categories